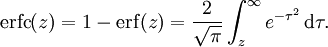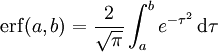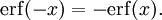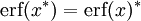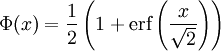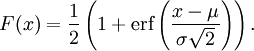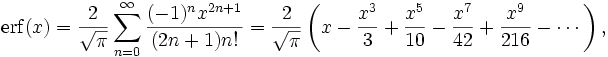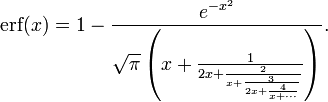- Error function
-
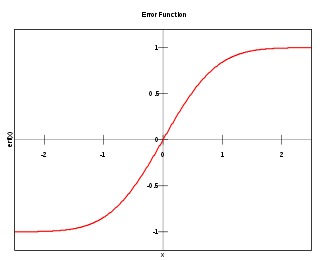
Als Fehlerfunktion bezeichnet man in der Approximationstheorie die Differenz zwischen einer Funktion und ihrer besten Approximation. In der Theorie der speziellen Funktionen versteht man unter Fehlerfunktion oder Gaußsche Fehlerfunktion das Integral
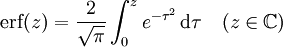 .
.
Die Fehlerfunktion findet Anwendung in der Statistik und in der Theorie der partiellen Differentialgleichungen.
Inhaltsverzeichnis
Eigenschaften
Die Bezeichnung erf(z) kommt von error function. Die komplementäre (bzw. konjugierte) Fehlerfunktion
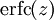 ist gegeben durch:
ist gegeben durch:Die imaginäre Fehlerfunktion
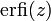 ist gegeben durch:
ist gegeben durch: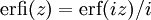 .
.
Die verallgemeinerte Fehlerfunktion
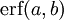 wird durch das Integral:
wird durch das Integral:definiert. Es gilt
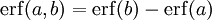 .
.Die Fehlerfunktion ist ungerade:
Weiterhin gilt für die Fehlerfunktion mit komplexem Argument x
wobei x * die komplexe Konjugation x darstellt.
Verwendung
Verwandtschaft mit der Normalverteilung
Die Fehlerfunktion hat eine gewisse Ähnlichkeit mit der Verteilungsfunktion der Normalverteilung. Sie hat jedoch eine Zielmenge von ( − 1,1), während eine Verteilungsfunktion zwingend Werte aus dem Bereich [0,1] annehmen muss.
Es gilt für die Standardnormalverteilung
bzw. für die Verteilungsfunktion F einer beliebigen Normalverteilung mit Standardabweichung σ und Erwartungswert μ
Wenn die Ergebnisse einer Messreihe durch eine Normalverteilung mit Standardabweichung σ und Erwartungswert 0 beschrieben werden können, dann ist
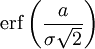 die Wahrscheinlichkeit, mit der der Messfehler einer einzelnen Messung zwischen − a und + a liegt.
die Wahrscheinlichkeit, mit der der Messfehler einer einzelnen Messung zwischen − a und + a liegt.Wärmeleitungsgleichung
Die Fehlerfunktion und die komplementäre Fehlerfunktion kommen beispielsweise in Lösungen der Wärmeleitungsgleichung vor, wenn Randwertbedingungen durch die Heaviside-Funktion vorgegebenen sind.
Numerische Berechnung
Die Fehlerfunktion ist wie die Verteilungsfunktion der Normalverteilung nicht durch eine geschlossene Funktion darstellbar und muss numerisch bestimmt werden.
Für kleine reelle Werte erfolgt die Berechnung mit der Reihenentwicklung
für große reelle Werte mit der Kettenbruchentwicklung
Wertetabelle
-
x erf(x) erfc(x) x erf(x) erfc(x) 0,00 0,0000000 1,0000000 1,30 0,9340079 0,0659921 0,05 0,0563720 0,9436280 1,40 0,9522851 0,0477149 0,10 0,1124629 0,8875371 1,50 0,9661051 0,0338949 0,15 0,1679960 0,8320040 1,60 0,9763484 0,0236516 0,20 0,2227026 0,7772974 1,70 0,9837905 0,0162095 0,25 0,2763264 0,7236736 1,80 0,9890905 0,0109095 0,30 0,3286268 0,6713732 1,90 0,9927904 0,0072096 0,35 0,3793821 0,6206179 2,00 0,9953223 0,0046777 0,40 0,4283924 0,5716076 2,10 0,9970205 0,0029795 0,45 0,4754817 0,5245183 2,20 0,9981372 0,0018628 0,50 0,5204999 0,4795001 2,30 0,9988568 0,0011432 0,55 0,5633234 0,4366766 2,40 0,9993115 0,0006885 0,60 0,6038561 0,3961439 2,50 0,9995930 0,0004070 0,65 0,6420293 0,3579707 2,60 0,9997640 0,0002360 0,70 0,6778012 0,3221988 2,70 0,9998657 0,0001343 0,75 0,7111556 0,2888444 2,80 0,9999250 0,0000750 0,80 0,7421010 0,2578990 2,90 0,9999589 0,0000411 0,85 0,7706681 0,2293319 3,0 0,9999779 0,0000221 0,90 0,7969082 0,2030918 3,10 0,9999884 0,0000116 0,95 0,8208908 0,1791092 3,20 0,9999940 0,0000060 1,00 0,8427008 0,1572992 3,30 0,9999969 0,0000031 1,10 0,8802051 0,1197949 3,40 0,9999985 0,0000015 1,20 0,9103140 0,0896860 3,50 0,9999993 0,0000007
Literatur
- Milton Abramowitz and Irene A. Stegun (Hrsg.): Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. New York: Dover, 1972. (siehe Chapter 7)
- William H. Press, Saul A. Teukolsky, William T. Vetterling und Brian P. Flannery: Numerical Recipes in C. 2. Auflage, Cambridge 1992. (S.220ff)
Wikimedia Foundation.