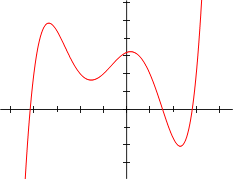
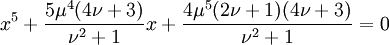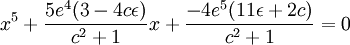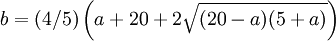Quintic equation

Eine Gleichung fünften Grades oder quintische Gleichung ist in der Mathematik ein Polynom vom Grad fünf. Es ist von der Form

ax⁵ + bx⁴ + cx³ + dx² + ex + f = 0,

wobei die Koeffizienten a,b,c,d,e und f Elemente eines Körpers (typischerweise die rationalen, reellen oder komplexen Zahlen), mit 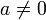$a \neq 0$ sind. Man spricht dann von einer Gleichung (oder einem Polynom) "über" diesem Körper.

Polynom vom Grad 5 : f(x) = (x+4)(x+2)(x+1)(x-1)(x-3)/20+2

Geschichte

Das Auflösen von Polynomgleichungen durch endliche Wurzelausdrücke (Radikale) ist ein altes Problem. Nachdem 1539 Gerolamo Cardano Lösungen für die allgemeinen Gleichungen bis zum Grad 4 veröffentlicht hatte, konzentrierten sich die Anstrengungen auf die Lösung der allgemeinen Gleichung fünften Grades. 1771 fand Gianfrancesco Malfatti als erster einen Lösungsweg, der allerdings nur im Fall der Auflösbarkeit durch Wurzelausdrücke funktioniert. Paolo Ruffini veröffentlichte 1799 einen lückenhaften Beweis für die Unauflösbarkeit der allgemeinen Gleichung 5. Grades. Da Ruffini für die damalige Zeit ungewohnte Argumente verwendete, die heute der Gruppentheorie zugeordnet werden, wurde sein Beweis zunächst nicht akzeptiert. 1824 gelang Niels Henrik Abel ein vollständiger Beweis dafür, dass die allgemeine Gleichung fünften Grades nicht durch Radikale auflösbar ist (Satz von Abel-Ruffini). In der Galoistheorie lässt sich der Beweis verkürzt so darstellen: Die Galoisgruppe der allgemeinen Gleichung n-ten Grades hat die Alternierende Gruppe A_n als Faktor, und diese Gruppe ist einfach für 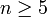$n \ge 5$ (vgl. Ikosaedergruppe), also nicht auflösbar. Charles Hermite gelang es 1858, die allgemeine Gleichung fünften Grades in jacobischen Thetafunktionen (aber natürlich nicht in Radikalen) zu lösen.

Lösbare Gleichungen fünften Grades

Manche Gleichungen fünften Grades können in Radikale faktorisiert werden, etwa x⁵ − x⁴ − x + 1 = 0, welches man zu (x² + 1)(x + 1)(x − 1)² = 0 umschreiben kann. Andere Gleichungen wie etwa x⁵ − x + 1 = 0 können nicht in dieser Form aufgelöst werden. Évariste Galois entwickelte um 1830 Techniken um zu bestimmen, ob eine gegebene Gleichung in Wurzeln lösbar ist, daraus entwickelte sich die Galoistheorie. Mit dieser konnten dann George Paxton Young^[1] und Carl Runge^[2] 1885 ein Kriterium erarbeiten, um zu bestimmen in welchen Fällen eine gegebene quintische Gleichung lösbar ist (Vgl. die Arbeit von Lazard für einen modernen Zugang). Sie zeigten, dass jede gebene irreduzible lösbare quintische Gleichung in Bring-Jerrard form,

x⁵ + ax + b = 0

von der Form

$x^5 + \frac{5\mu^4(4\nu + 3)}{\nu^2 + 1}x + \frac{4\mu^5(2\nu + 1)(4\nu + 3)}{\nu^2 + 1} = 0$

mit rationalem μ und ν sein muss. Im Jahre 1994 fanden Blair Spearman and Kenneth S. Williams die Darstellung

$x^5 + \frac{5e^4(3-4c\epsilon)}{c^2 + 1}x + \frac{-4e^5(11\epsilon+2c)}{c^2 + 1} = 0$

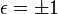für $\epsilon = \pm 1$. Die Beziehung zwischen den beiden Parametrisierungen kann durch die Gleichung

$b = (4/5)\left(a+20+2\sqrt{(20-a)(5+a)}\right)$

mit

$a = \frac{5(4v+3)}{v^2+1}$

hergestellt werden. Im Fall der negativen Quadratwurzel erhält man bei geeigneter Skalierung den erste Parametrisierung, bei positiver Quadratwurzel die zweite mit ε = − 1. Daher ist es eine notwendige (aber keine hinreichende) Bedingung für eine lösbare Gleichung fünften Grades der Form

z⁵ + aμ⁴z + bμ⁵ = 0

mit rationalem a,b und μ, dass die Gleichung

y² = (20 − a)(5 + a)

eine rationale Lösung y hat.

Mit Hilfe von Tschirnhaus-Transformationen ist es möglich, jede Gleichung fünften Grades in Bring-Jerrard Form zu bringen, daher geben sowohl die Parametrisierungen von Runge und Young als auch von Spearman und Williams notwendige und hinreichende Bedingungen um zu prüfen, ob eine beliebige quintische Gleichung in Radikalen zu lösen ist.

Beispiele für lösbare Gleichungen fünften Grades

Eine Gleichung ist in Radikalen lösbar, wenn ihre Galoisgruppe eine auflösbare Gruppe ist. Für Gleichungen nten Grades ist ihre Galoisgruppe eine Untergruppe der Symmetrischen Gruppe S_n, der Permutationen von n Elementen.

Ein einfaches Beispiel für eine lösbare Gleichung ist x⁵ − 5x⁴ − 10x³ − 10x² − 5x − 1 = 0 mit der Galoisgruppe F(5) die von den Permutationen "(1 2 3 4 5)" und "(1 2 4 3)" erzeugt wird; die einzige reelle Wurzel ist $x=1+\sqrt[5]{2}+\sqrt[5]{4}+\sqrt[5]{8}+\sqrt[5]{16}.$

Allerdings können die Lösungen auch wesentlich komplexer sein. Zum Beispiel hat die Gleichung x⁵ − 5x + 12 = 0 die Galoisgruppe D(5) welche von "(1 2 3 4 5)" und "(1 4)(2 3)" erzeugt wird, und die Lösung benötigt ausgeschrieben etwa 600 Symbole.

Literatur

• Charles Hermite: Sur la résolution de l'équation du cinquième degré. In: Œuvres de Charles Hermite. Band 2, Seiten 5-21, Gauthier-Villars, 1908 (online verfügbar).
• Felix Klein: Vorlesungen über das Ikosaeder und die Auflösung der Gleichungen vom fünften Grade. Leipzig 1884, ISBN 0-486-49528-0 (online verfügbar).
• Leopold Kronecker: Sur la résolution de l'equation du cinquième degré, extrait d'une lettre adressée à M. Hermite. In: Comptes Rendus de l'Académie des Sciences. Band 66 Nr. 1, 1858, Seiten 1150-1152.
• Blair Spearman und Kenneth S. Williams: Characterization of solvable quintics x⁵ + ax + b. In: American Mathematical Monthly. Band 101, 1994, Seiten 986-992.
• Ian Stewart: Galois Theory. 2. Auflage. Chapman and Hall, 1989, ISBN 0-412-34550-1.
• Jörg Bewersdorff: Algebra für Einsteiger: Von der Gleichungsauflösung zur Galois-Theorie. Vieweg, 2007, ISBN 978-3-8348-0095-4. Kapitel 8 beschreibt die Lösung lösbarer Gleichungen fünften Grades in der Form x⁵ + cx + d = 0 (Buchkapitel in englischer Übersetzung The solution of equations of the fifth degree ist online verfügbar).
• Victor S. Adamchik und David J. Jeffrey: Polynomial transformations of Tschirnhaus, Bring and Jerrard. In: ACM SIGSAM Bulletin. Band 37 Nr. 3, September 2003, Seiten 90-94.
• Ehrenfried Walter von Tschirnhaus: A method for removing all intermediate terms from a given equation. In: ACM SIGSAM Bulletin. Band 37 Nr. 1, März 2003, Seiten 1-3.
• Daniel Lazard: Solving quintics in radicals. In: Olav Arnfinn Laudal, Ragni Piene: The Legacy of Niels Henrik Abel. Berlin 2004, Seiten 207–225, ISBN 3-5404-3826-2.
• Peter Pesic: Abels Beweis. Springer 2005, ISBN 3-540-22285-5.
• Jean-Pierre Tignol: Galois' Theory of Algebraic Equations. World Scientific, 2004, ISBN 981-02-4541-6.

Einzelnachweise

1. ↑ Young, G. P.: Solution of Solvable Irreducible Quintic Equations, Without the Aid of a Resolvent Sextic. In: Amer. J. Math. Band 7, Seiten 170-177, 1885.
2. ↑ Runge, C.: Über die auflösbaren Gleichungen von der Form x⁵ + ux + v = 0. In: Acta Math. Band 7, Seiten 173-186, 1885.

Siehe auch:

• Auflösbare Gruppe
• Quartische Gleichung
• Lösen von Gleichungen

Weblinks

• Mathworld - Quintic Equation - weitere Details über Methoden zur Lösung von Gleichungen fünften Grades.
• Abels und Galois' Aufsätze - die Originale sind online verfügbar.
• Solving the Quintic with Mathematica - Poster zur Gleichung fünften Grades.
• Solving Solvable Quintics - eine Methode zur Lösung lösbarer Gleichungen fünften Grades nach David S. Dummit.
• Polynomial Transformations of Tschirnhaus, Bring and Jerrard - ein Aufsatz von Victor S. Adamchik & David J. Jeffrey zu Polynomtransformationen.