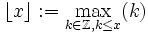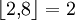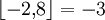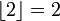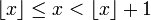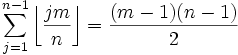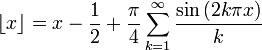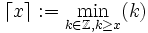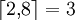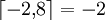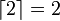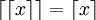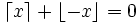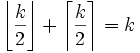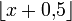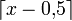- Gaussklammer
-
Die Gaußklammer oder Abrundungsfunktion (auch Ganzzahl-Funktion, Ganzteilfunktion oder Entier-Klammer; engl. floor function) und die Aufrundungsfunktion (engl. ceiling function) sind Funktionen, die einer reellen Zahlen die nächstgrößere bzw. nächstkleinere ganze Zahl zuordnen. Die Notation wurde nach Carl Friedrich Gauß benannt, der das Symbol
![\left[ x \right]](/pictures/dewiki/52/4fcbf9b418b2ad4ef36169fb15a32bdd.png) für die Abrundungsfunktion 1808 eingeführt hat[1]. Ende des 20. Jahrhunderts verbreiteten sich auch die von Kenneth E. Iverson eingeführten Bezeichnungen
für die Abrundungsfunktion 1808 eingeführt hat[1]. Ende des 20. Jahrhunderts verbreiteten sich auch die von Kenneth E. Iverson eingeführten Bezeichnungen 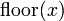 und
und 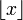 für die Gaußklammer sowie
für die Gaußklammer sowie 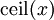 und
und 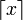 für die Aufrundungsfunktion[2].
für die Aufrundungsfunktion[2].Inhaltsverzeichnis
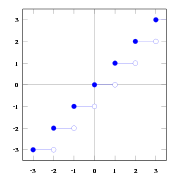
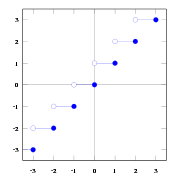
Die Abrundungsfunktion oder Gaußklammer
Definition
Sie ist folgendermaßen definiert:
- Für eine reelle Zahl x ist
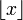 die größte ganze Zahl, die kleiner oder gleich x ist:
die größte ganze Zahl, die kleiner oder gleich x ist:
Beispiele
-
- Das Ergebnis ist nicht, wie vielleicht vermutet, − 2, da definitionsgemäß gelten muss:
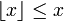 und − 2 dieser Definition nicht gerecht wird.
und − 2 dieser Definition nicht gerecht wird.
- Das Ergebnis ist nicht, wie vielleicht vermutet, − 2, da definitionsgemäß gelten muss:
Eigenschaften
- Es gilt immer
Dabei ist
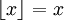 genau dann, wenn x eine ganze Zahl ist.
genau dann, wenn x eine ganze Zahl ist.- Für jede ganze Zahl k und jede reelle Zahl x gilt
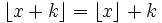 .
.
- Für positive reelle Zahlen x,y gilt
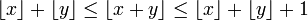 .
.
- Für positive reelle Zahlen n,x gilt:
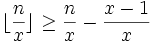 .
.
- Es gilt
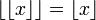 .
.
- Sind m und n teilerfremde natürliche Zahlen, dann gilt
- Die Ganzzahl-Funktion ist nicht stetig, aber oberhalbstetig.
- für nichtganze reelle x lässt sie sich folgendermaßen als Fourier-Reihe darstellen:
Aufrundungsfunktion
Definition
Sie ist so definiert:
- Für eine reelle Zahl x ist
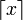 die kleinste ganze Zahl, die größer oder gleich x ist.
die kleinste ganze Zahl, die größer oder gleich x ist.
Beispiele
Eigenschaften
- Es gilt analog
Allgemeine Eigenschaften
Zusammenhänge zwischen Auf- und Abrundungsfunktion
- Es ist stets
- Für ganze Zahlen k gilt:
Gewöhnliche Rundung
Die gewöhnliche Rundung auf die nächstliegende ganze Zahl kann auch mit diesen Funktionen ausgedrückt werden:
Einzelnachweise
- ↑ Earliest Uses of Function Symbols, 21. Juni 2007: Until recently [x] has been the standard symbol for the greatest integer function. According to Grinstein (1970), "The use of the bracket notation, which has led some authors to term this the bracket function, stems back to the work of Gauss (1808) in number theory. The function is also referred to by Legendre who used the now obsolete notation E(x)." The Gauss reference is to Theorematis arithmetici demonstratio nova. Werke Volume: Bd. 2 p. 5.
- ↑ Earliest Known Uses of Some of the Words of Mathematics (C), 21. Juni 2007: The terms CEILING FUNCTION and FLOOR FUNCTION appear in Kenneth E. Iverson's A Programming Language (1962, p. 12): "Two functions are defined: 1. the floor of x (or integral part of x) denoted by
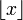 and defined as the largest integer not exceeding x, 2. the ceiling of x denoted by
and defined as the largest integer not exceeding x, 2. the ceiling of x denoted by 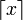 and defined as the smallest integer not exceeded by x." This was the first appearance of the terms and symbols, according to R. L. Graham, D. E. Knuth & O. Patashnik Concrete Mathematics: A Foundation for Computer Science (1989, p. 67).
and defined as the smallest integer not exceeded by x." This was the first appearance of the terms and symbols, according to R. L. Graham, D. E. Knuth & O. Patashnik Concrete Mathematics: A Foundation for Computer Science (1989, p. 67).
- Für eine reelle Zahl x ist
Wikimedia Foundation.