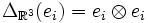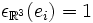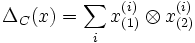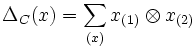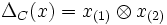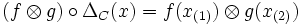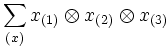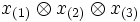- Sweedler Notation
-
Koalgebra berührt die Spezialgebiete
ist Spezialfall von
ist dual zu
- unitäre assoziative Algebra
umfasst als Spezialfälle
Eine Koalgebra ist ein Vektorraum, der die zu einer Algebra duale Struktur besitzt. Das heißt anstelle einer Multiplikation, die zwei Elemente auf ihr Produkt abbildet, gibt es eine Komultiplikation, die ein Element auf ein Tensorprodukt abbildet, und anstelle eines neutralen Elements, das die Einbettung des Grundkörpers in die Algebra ermöglicht, gibt es eine Abbildung aus der Koalgebra in den Grundkörper, die Koeins genannt wird.
Inhaltsverzeichnis
Definition
Eine Koalgebra über einem Körper k ist ein k-Vektorraum C mit Vektorraumhomomorphismen
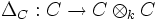 , genannt Komultiplikation, Koprodukt oder auch Diagonale, und
, genannt Komultiplikation, Koprodukt oder auch Diagonale, und  , genannt Koeins, so dass
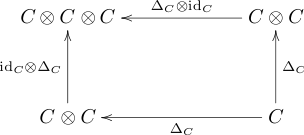
, genannt Koeins, so dass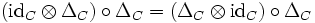 (Koassoziativität)
(Koassoziativität)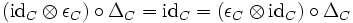 (Koeins)
(Koeins)
Ein Koalgebrahomomorphismus zwischen zwei Koalgebren C und D ist ein Vektorraumhomomorphismus
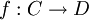 mit
mit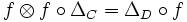 und
und 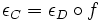 .
.
Beispiel
Sei (e1,e2,e3) die kanonische Basis von
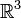 . Man kann auf
. Man kann auf 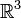 eine Koalgebra-Struktur mittels
eine Koalgebra-Struktur mittelsund
definieren.
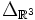 ist koassoziativ, da
ist koassoziativ, da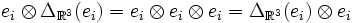 ,
,
und
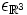 ist Koeins, da
ist Koeins, da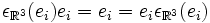 .
.
Die Elemente von
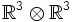 sind Tensoren zweiter Stufe und können daher als Matrizen dargestellt werden. Die Komultiplikation ist dann
sind Tensoren zweiter Stufe und können daher als Matrizen dargestellt werden. Die Komultiplikation ist dann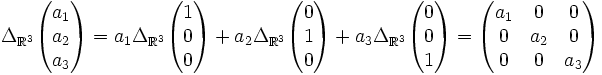 .
.
Dualität
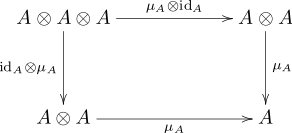
Die Multiplikation μA einer (unitären assoziativen) Algebra A ist bilinear, und aufgrund der Universellen Eigenschaft des Tensorprodukts kann sie als Abbildung von
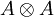 nach A aufgefasst werden. Die Multiplikation ist genau dann assoziativ, wenn das folgende Diagramm kommutiert.
nach A aufgefasst werden. Die Multiplikation ist genau dann assoziativ, wenn das folgende Diagramm kommutiert.Eine Algebra A besitzt genau dann ein neutrales Element, wenn es einen Vektorraumhomomorphismus ηA gibt, so dass das folgende Diagramm kommutiert:
In diesem Fall gilt 1A = ηA(1k).
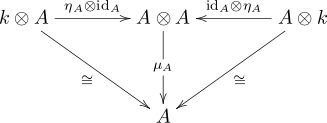
Eine Koalgebra C ist eine Algebra in der zu den Vektorräumen Vekt dualen Kategorie Vektop. Das heißt, anstelle der Multiplikation gibt es eine Abbildung von
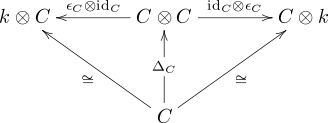
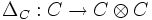 , so dass das folgende duale Diagramm kommutiert:
, so dass das folgende duale Diagramm kommutiert:Und anstelle eines neutralen Elements gibt es eine Abbildung
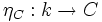 , so dass das folgende duale Diagramm kommutiert:
, so dass das folgende duale Diagramm kommutiert:Sweedler Notation
Über das Koprodukt ΔC(x) eines Elements
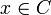 ist im Allgemeinen nur bekannt, dass es in
ist im Allgemeinen nur bekannt, dass es in 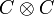 liegt und sich folglich als
liegt und sich folglich alsdarstellen lässt. In der Sweedler-Notation wird dies abgekürzt, indem man symbolisch
schreibt. In summenloser Sweedler-Notation verzichtet man sogar auf das Summensymbol und schreibt
Es ist dabei wichtig zu beachten, dass diese Schreibweise nach wie vor eine Summe bezeichnet. Die Symbole x(1) und x(2) sind für sich allein bedeutungslos und stehen nicht für bestimmte Elemente aus C.
Diese Schreibweise ermöglicht es, die Komposition von ΔC mit anderen Funktionen als
zu schreiben.
In summenloser Sweedler-Notation ist εC genau dann Koeins, wenn
- εC(x(1))x(2) = x = x(1)εC(x(2)).
Das Koprodukt ΔC ist genau dann koassoziativ, wenn
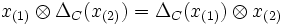 .
.
Dieses Element wird in Sweedler-Notation symbolisch als
und summenlos als
geschrieben.
Durch erneutes Anwenden von ΔC entstehen längere Tensorprodukte, die analog geschrieben werden. Dabei muss man die „Indizes“ der hinteren Elemente gegebenenfalls erhöhen:
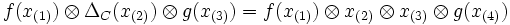 .
.
Durch Anwenden von εC verkürzen sich die Tensorprodukte, die „Indizes“ der hinteren Elemente werden entsprechend angepasst:
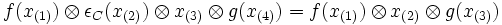 .
.
Literatur
- Christian Kassel: Quantum Groups In: Graduate Texts in Mathematics. Springer-Verlag, ISBN 0-387-94370-6.
Wikimedia Foundation.