Bialgebra

Bialgebra
berührt die Spezialgebiete
Mathematik Abstrakte Algebra Lineare Algebra Kommutative Algebra
ist Spezialfall von
Algebra Koalgebra
umfasst als Spezialfälle
Hopf-Algebra

Eine Bialgebra hat sowohl die Struktur einer unitären, assoziativen Algebra als auch die dazu duale Struktur einer Koalgebra. Der wichtigste Spezialfall von Bialgebren sind Hopf-Algebren, zu denen auch die Quantengruppen gehören.

Definition

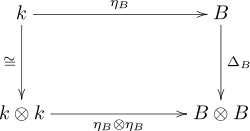
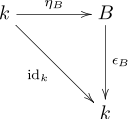
Sei k ein Körper und B sowohl unitäre assoziative Algebra über k als auch Koalgebra über k. Dabei bezeichne μ_B die Multiplikation, η_B die Eins (Einbettung des Körpers in die Algebra), Δ_B die Komultiplikation und $\epsilon_B$ die Koeins.

B heißt Bialgebra über k wenn die folgenden äquivalenten Kompatibilitätsbedingungen erfüllt sind.

• Die Komultiplikation Δ_B und die Koeins $\epsilon_B$ sind Algebrahomomorphismen.
• Die Multiplikation μ_B und die Eins η_B sind Koalgebrahomomorphismen.
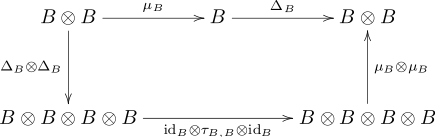
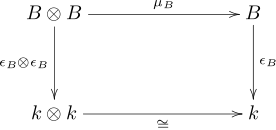
• Die folgenden Diagramme kommutieren

Dabei ist $\tau_{B,B}:=v\otimes w\mapsto w\otimes v$ die "Flip"-Abbildung, also der kanonische Isomorphismus der Tensorprodukte $V\otimes W$ und $W\otimes V$ angewandt auf $B\otimes B$.

Die Bialgebren bilden zusammen mit den Abbildungen, die sowohl Algebra- als auch Koalgebrahomomorphismen sind, eine Kategorie.

Verallgemeinerung

Algebren und Koalgebren können in beliebigen monoidalen Kategorien betrachtet werden. Für Kompatibilitätsbedingungen ist es jedoch notwendig, dass auch das Tensorprodukt einer (Ko)Algebra auf natürliche Weise wieder eine (Ko)Algebra ist, dies bedingt die Existenz einer Zopfung.

Literatur

• Christian Kassel: Quantum Groups (Graduate Texts in Mathematics). Springer-Verlag, ISBN 0-387-94370-6