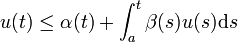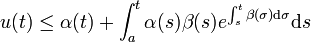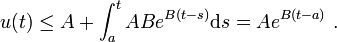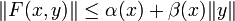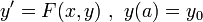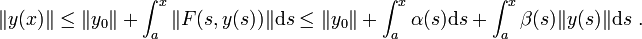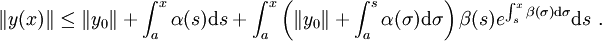- Gronwall-Lemma
-
Die grönwallsche Ungleichung ist eine Ungleichung, die es erlaubt, aus der impliziten Information einer Integralungleichung explizite Schranken herzuleiten. Des Weiteren ist sie ein wichtiges Hilfsmittel zum Beweis von Existenz- und Einschließungssätzen für Lösungen von Differential- und Integralgleichungen. Sie ist nach Thomas Hakon Grönwall benannt, der sie im Jahr 1919 bewies und in einer wissenschaftlichen Veröffentlichung beschrieb.
Inhaltsverzeichnis
Formulierung
Gegeben seien ein Intervall
![\ I := [a, b]](/pictures/dewiki/57/96379f8ef2d1808ebaf687392c67b128.png) sowie stetige Funktionen
sowie stetige Funktionen 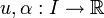 und
und 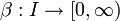 . Weiter gelte die Integralungleichung
. Weiter gelte die Integralungleichungfür alle
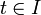 . Dann gilt die grönwallsche Ungleichung
. Dann gilt die grönwallsche Ungleichungfür alle
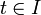 .
.Spezialfall
Im Fall konstanter Funktionen
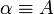 und
und 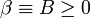 lautet die grönwallsche Ungleichung
lautet die grönwallsche UngleichungAnwendungen
Eindeutigkeitssatz für Anfangswertprobleme
Es sei
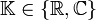 ,
, 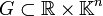 ,
, 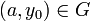 und
und 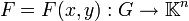 stetig sowie lokal Lipschitz-stetig bezüglich der zweiten Variablen. Dann besitzt das Anfangswertproblem
stetig sowie lokal Lipschitz-stetig bezüglich der zweiten Variablen. Dann besitzt das Anfangswertproblem 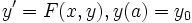 höchstens eine Lösung
höchstens eine Lösung  .
.Linear beschränkte Differentialgleichungen
Seien
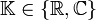 ,
, 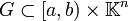 ,
, 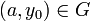 ,
, 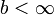 und
und 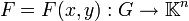 stetig. Weiter gebe es Funktionen
stetig. Weiter gebe es Funktionen 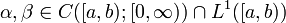 derart, dass
derart, dassfür alle
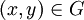 . Dann gilt für jede Lösung y von
. Dann gilt für jede Lösung y vonauf [a,b), dass y beschränkt ist.
Beweis
Es gilt
Die grönwallsche Ungleichung impliziert

Weblinks
- Beweis der grönwallschen Ungleichung im Beweisarchiv
- Anwendung der grönwallschen Ungleichung für den Eindeutigkeitssatz von Anfangswertproblemen bei lokal Lipschitz-stetiger Differentialgleichung
- Die grönwallsche Ungleichung auf planetmath.org
Literatur
- Herbert Amann: Gewöhnliche Differentialgleichungen. 2. Auflage. Gruyter - de Gruyter Lehrbücher, Berlin/New York 1995, ISBN 3-11-014582-0.
Wikimedia Foundation.