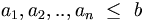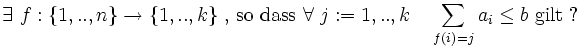Bin packing problem — In computational complexity theory, the bin packing problem is a combinatorial NP hard problem. In it, objects of different volumes must be packed into a finite number of bins of capacity V in a way that minimizes the number of bins used.There… … Wikipedia
Bin Packing Problem — Das Behälterproblem oder auch Bin Packing ist ein kombinatorisches Optimierungsproblem, das auf folgender Fragestellung basiert: Gegeben: Eine Anzahl von „Behältern“ (englisch bin) der Größe und eine Anzahl „Objekte“ mit den Größen … Deutsch Wikipedia
Bin packing problem — Problème de bin packing Le problème de bin packing relève de la recherche opérationnelle et de l optimisation combinatoire. Il s agit de trouver le rangement le plus économique possible pour un ensemble d articles dans des boîtes. Le problème… … Wikipédia en Français
Packing problem — Part of a series on Puzzles … Wikipedia
BIN PACKING — Das Behälterproblem oder auch Bin Packing ist ein kombinatorisches Optimierungsproblem, das auf folgender Fragestellung basiert: Gegeben: Eine Anzahl von „Behältern“ (englisch bin) der Größe und eine Anzahl „Objekte“ mit den Größen … Deutsch Wikipedia
Bin-Packing — Das Behälterproblem oder auch Bin Packing ist ein kombinatorisches Optimierungsproblem, das auf folgender Fragestellung basiert: Gegeben: Eine Anzahl von „Behältern“ (englisch bin) der Größe und eine Anzahl „Objekte“ mit den Größen … Deutsch Wikipedia
Bin-packing — Das Behälterproblem oder auch Bin Packing ist ein kombinatorisches Optimierungsproblem, das auf folgender Fragestellung basiert: Gegeben: Eine Anzahl von „Behältern“ (englisch bin) der Größe und eine Anzahl „Objekte“ mit den Größen … Deutsch Wikipedia
Probleme de bin packing — Problème de bin packing Le problème de bin packing relève de la recherche opérationnelle et de l optimisation combinatoire. Il s agit de trouver le rangement le plus économique possible pour un ensemble d articles dans des boîtes. Le problème… … Wikipédia en Français
Problème de bin packing — Le problème de bin packing relève de la recherche opérationnelle et de l optimisation combinatoire. Il s agit de trouver le rangement le plus économique possible pour un ensemble d articles dans des boîtes. Le problème classique se définit en une … Wikipédia en Français
Knapsack problem — BKP redirects here. For other uses, see BKP (disambiguation). Example of a one dimensional (constraint) knapsack problem: which boxes should be chosen to maximize the amount of money while still keeping the overall weight under or equal to… … Wikipedia
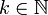 und eine Anzahl
und eine Anzahl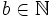 „Objekte“ mit den Größen
„Objekte“ mit den Größen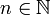 .
.