Gauss-Elimination

Das gaußsche Eliminationsverfahren oder einfach Gauß-Verfahren (nach Carl Friedrich Gauß) ist ein Algorithmus aus den mathematischen Teilgebieten der linearen Algebra und der Numerik. Es ist ein wichtiges Verfahren zum Lösen von linearen Gleichungssystemen und beruht darauf, dass elementare Umformungen zwar das Gleichungssystem ändern, aber die Lösung erhalten. Dies erlaubt es, jedes Gleichungssystem auf Stufenform zu bringen, an der die Lösung durch sukzessive Elimination der Unbekannten leicht ermittelt oder die Lösungsmenge abgelesen werden kann.

Die Anzahl der benötigten Operationen ist bei einer 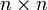$n\times n$-Matrix von der Größenordnung n³. In seiner Grundform ist der Algorithmus anfällig für Rundungsfehler, aber mit kleinen Modifikationen (Pivotisierung) stellt er für allgemeine lineare Gleichungssysteme das Standardlösungsverfahren dar und ist Teil aller wesentlichen Programmbibliotheken für numerische lineare Algebra wie NAG, IMSL und LAPACK.