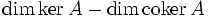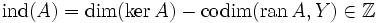Fredholm-Index

In der Funktionalanalysis, einem Teilgebiet der Mathematik, ist der Begriff des Fredholm-Operators (nach E. I. Fredholm) eine Verallgemeinerung der Invertierbarkeit einer linearen Abbildung zwischen Vektorräumen. Für Fredholm-Operatoren kann der Fredholm-Index

$\dim\ker A-\dim\mathrm{coker}\,A$

definiert werden, der ein Spezialfall der Euler-Charakteristik ist.

Definition

Ein beschränkter linearer Operator 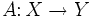$A\colon X\to Y$ zwischen zwei Banachräumen X und Y heißt ein Fredholm-Operator, oder man sagt kurz: "A ist Fredholm", wenn

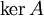• $\ker A$ endliche Dimension hat und
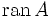• $\mathrm{ran}\,A$ endliche Kodimension in Y hat.

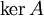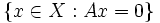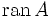Dabei ist $\ker A$ der Kern von A, also die Menge $\{x\in X: Ax=0\}$ und $\mathrm{ran}\,A$ ist das Bild von A, also die Teilmenge $\{Ax\mid x\in X\}\subseteq Y$.

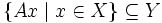Die Zahl

$\mathrm{ind}(A)= \dim(\ker A) - \mathrm{codim}(\mathrm{ran}\,A,Y)\in\mathbb Z$

heißt Fredholm-Index von A.

Eigenschaften

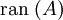• $\mathrm{ran}\; (A)$ ist ein abgeschlossener Unterraum.
• Die Abbildung

$\mathrm{ind}\colon A\mapsto\mathrm{ind}(A)$

ist stetig bezüglich der Operatornorm und daher wegen der Diskretheit von 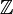$\mathbb{Z}$ konstant auf Zusammenhangskomponenten.

• Ein Operator 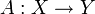$A: X\to Y$ ist genau dann ein Fredholm-Operator, wenn es Operatoren B₁,B₂ und kompakte Operatoren K₁,K₂ gibt, so dass AB₁ = I_Y − K₁ und B₂A = I_X − K₂ gilt, d.h. wenn A modulo kompakter Operatoren invertierbar ist. 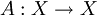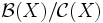Insbesondere ist ein beschränkter Operator $A: X\to X$ genau dann ein Fredholm Operator, wenn seine Klasse $[A]_{\mathcal{C}(X)}$ in der Calkin-Algebra $\mathcal{B}(X)/\mathcal{C}(X)$ invertierbar ist.
• Für jeden Fredholm-Operator A und jeden kompakten Operator K ist A + K ebenfalls ein Fredholm-Operator mit selbem Fredholm-Index wie A. Insbesondere ist jede kompakte Störung der Identität, also jeder Operator der Form I + K für einen kompakten Operator K ein Fredholm-Operator vom Index 0.
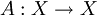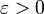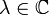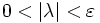• Ist $A: X\to X$ ein Fredholm-Operator, dann gibt es ein $\varepsilon > 0$, so dass für alle $\lambda\in\mathbb{C}$ mit $0 < |\lambda| < \varepsilon$ gilt

(i) A − λI ist ein Fredholm-Operator;
(ii)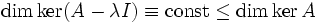 $\dim\ker (A - \lambda I) \equiv \mathrm{const} \le \dim\ker A$;
(iii)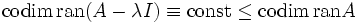 $\mathrm{codim}\,\mathrm{ran}(A - \lambda I) \equiv \mathrm{const} \le \mathrm{codim}\,\mathrm{ran}A$;
(iv) ind(A − λI) = ind(A).
Dieser Satz heißt auch das Punctured Neighbourhood Theorem.