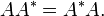Hyponormal

In der Funktionalanalysis verallgemeinert der normale Operator den Begriff der normalen Matrix aus der linearen Algebra. Ist X ein Hilbertraum, so heißt ein Operator 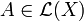$A \in \mathcal{L}(X)$ normal, falls er mit seiner Adjungierten 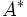$A^{\ast}$ kommutiert, d.h. wenn

$A A^{\ast} = A^{\ast} A.$

Dabei bezeichnet 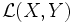$\mathcal{L}(X,Y)$ die Menge aller stetigen linearen Abbildungen von X nach Y und 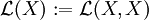$\mathcal{L}(X):=\mathcal{L}(X,X)$ die Menge der stetigen Endomorphismen von X.

Eigenschaften

Sei 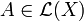$A\in\mathcal{L}(X)$ ein normaler Operator. Dann gilt:

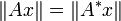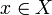
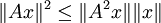• $\|Ax\| = \|A^{\ast}x\|$ für alle $x\in X$
• $\|Ax\|^2 \le \|A^2 x\| \|x\|$ für alle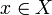 $x\in X$
• Operatornorm = Spektralradius, d.h.: $\|A\| = \sup\{|\lambda|; \lambda \in \sigma(A)\}$
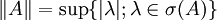
• Die von A erzeugte C^*-Algebra und die von A erzeugte Von-Neumann-Algebra sind kommutativ. Dieser Sachverhalt ermöglicht einen Funktionalkalkül.
• Die Diagonalisierbarkeit normaler Matrizen in der linearen Algebra verallgemeinert sich auf normale Operatoren in Form des Spektralsatzes.
• Eine Klassifikation normaler Operatoren besteht bzgl. unitärer Äquivalenz modulo kompakter Operatoren, indem man zur Calkin-Algebra übergeht, die im endlich-dimensionalen Fall {0} ist. Das ist im Artikel zur Calkin-Algebra ausgeführt.

Verwandte Begriffe

Ein Operator 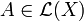$A\in\mathcal{L}(X)$ heißt

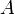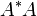• quasinormal, falls $A\,\!$ mit $A^{\ast}A$ vertauscht, das heißt $AA^{\ast}A=A^{\ast}AA$.
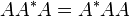• subnormal, falls es einen Hilbertraum Y gibt, so dass X unterraum von Y ist, und einen normalen Operator 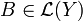$B\in\mathcal{L}(Y)$, so dass 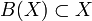$B(X)\subset X$ und $A=B|_X\,\!$
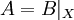
• hyponormal, falls 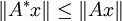$\|A^{\ast}x\| \le \|Ax\|$ für alle $x\in X$.
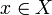• paranormal, falls 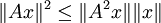$\|Ax\|^2 \le \|A^2x\| \|x\|$ für alle $x\in X$.
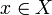• normaloid, falls Operatornorm = Spektralradius, d.h.: $\|A\| = \sup\{|\lambda|; \lambda \in \sigma(A)\}$.

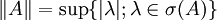Es gelten folgende Implikationen:

normal 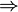$\Rightarrow$ quasinormal $\Rightarrow$ subnormal $\Rightarrow$ hyponormal $\Rightarrow$ paranormal $\Rightarrow$ normaloid.

Literatur

• Harro Heuser: Funktionalanalysis. B.G. Teubner, Stuttgart (1986), 3-519-22206-X.