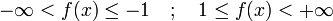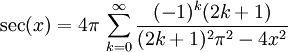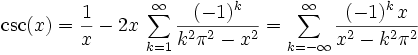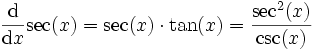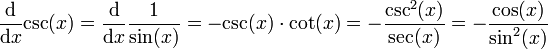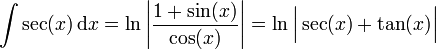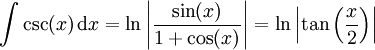- Secans
-
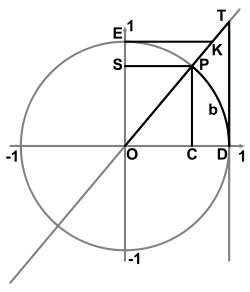
Sekans und Kosekans sind trigonometrische Funktionen. Der Sekans wird mit sec(x) bezeichnet, der Kosekans mit csc(x). Die Funktionen haben ihren Namen durch die Definition im Einheitskreis. Die Funktionswerte entsprechen der Länge von Sekantenabschnitten:
Im rechtwinkligen Dreieck ist der Sekans das Verhältnis der Hypotenuse zur Ankathete und damit die Kehrwertfunktion der Kosinusfunktion.
Der Kosekans ist das Verhältnis der Hypotenuse zur Gegenkathete und damit die Kehrwertfunktion der Sinusfunktion:
Inhaltsverzeichnis
Eigenschaften
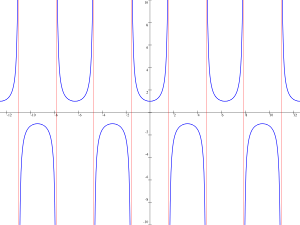
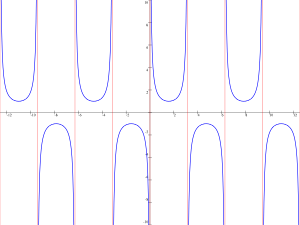
Graphen
Definitionsbereich
-
Sekans: 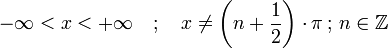
Kosekans: 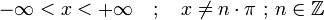
Wertebereich
Periodizität
- Periodenlänge
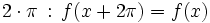
Symmetrien
-
Sekans: Gerade Funktion: f(x) = f( − x) Kosekans: Ungerade Funktion: f( − x) = − f(x)
Polstellen
-
Sekans: 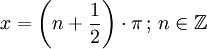
Kosekans: 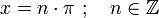
Extremwerte
-
Sekans: Minima: 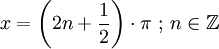
Maxima: 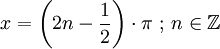
Kosekans: Minima: 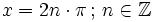
Maxima: 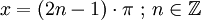
Weder die Sekansfunktion noch die Kosekansfunktion haben horizontale Asymptoten, Sprungstellen, Wendepunkte oder Nullstellen.
Umkehrfunktionen
Sekans:
- Auf einer halben Periodenlänge, z. B.
![x \in [0 , \pi]](/pictures/dewiki/101/ed0d5fb967bb60be6166d88803441d2f.png) ist die Funktion umkehrbar (Arkussekans):
ist die Funktion umkehrbar (Arkussekans): 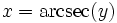
Kosekans
- Auf einer halben Periodenlänge, z. B.
![x \in \left[-\frac{\pi}{2} , \frac{\pi}{2} \right]](/pictures/dewiki/52/48af4ce3aea5ab5bbfdca2a3cb0786e2.png) ist die Funktion umkehrbar (Arkuskosekans):
ist die Funktion umkehrbar (Arkuskosekans): 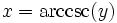
Reihenentwicklung
Sekans:
Kosekans:
Ableitung
Sekans:
Kosekans
Integral
Sekans:
Kosekans
Komplexes Argument
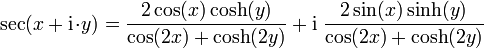 mit
mit 
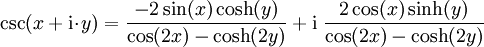 mit
mit 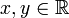
Siehe auch
Weblinks
Primäre trigonometrische Funktionen
Sinus und Kosinus | Tangens und Kotangens | Sekans und KosekansUmkehrfunktionen (Arkusfunktionen)
Arkussinus und Arkuskosinus | Arkustangens und Arkuskotangens | Arkussekans und ArkuskosekansHyperbelfunktionen
Sinus Hyperbolicus und Kosinus Hyperbolicus | Tangens Hyperbolicus und Kotangens Hyperbolicus | Sekans Hyperbolicus und Kosekans HyperbolicusAreafunktionen
Areasinus Hyperbolicus und Areakosinus Hyperbolicus | Areatangens Hyperbolicus und Areakotangens Hyperbolicus | Areasekans Hyperbolicus und Areakosekans Hyperbolicus
Wikimedia Foundation.