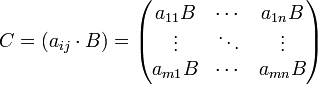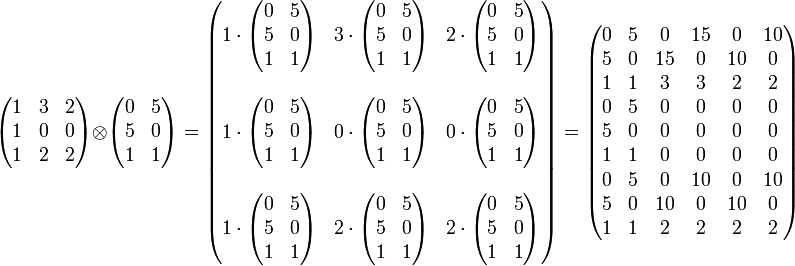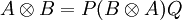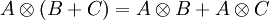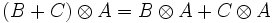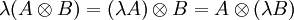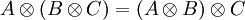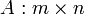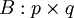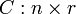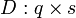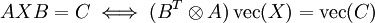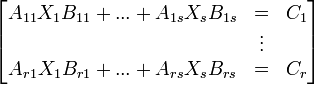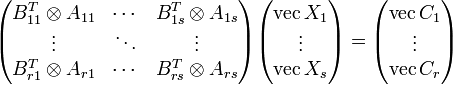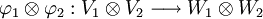Kroneckerprodukt

Das Kronecker-Produkt (nach Leopold Kronecker) ist ein Begriff aus der Matrizenrechnung.

Definition

Ist A eine 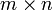$m\times n$ Matrix und B eine 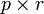$p\times r$ Matrix so ist das Kronecker-Produkt 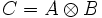$C = A \otimes B$ definiert als

$C = (a_{ij} \cdot B) =\begin{pmatrix} a_{11} B & \cdots & a_{1n} B \\ \vdots & \ddots & \vdots \\ a_{m1} B & \cdots & a_{mn} B \end{pmatrix}$

Das heißt jedes Element der Matrix A wird mit der Matrix B multipliziert. Das Ergebnis ist also wieder eine Matrix, allerdings von der Dimension $mp\times nr$.

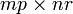1. Beispiel

$\begin{pmatrix} 1 & 2 \\ 3 & 4 \end{pmatrix}\otimes \begin{pmatrix} 5 & 6 \\ 7 & 8 \end{pmatrix} =\begin{pmatrix} 1\cdot \begin{pmatrix} 5 & 6 \\ 7 & 8 \end{pmatrix} & 2 \cdot \begin{pmatrix} 5 & 6 \\ 7 & 8 \end{pmatrix}\\\\ 3\cdot \begin{pmatrix} 5 & 6 \\ 7 & 8 \end{pmatrix} & 4 \cdot \begin{pmatrix} 5 & 6 \\ 7 & 8 \end{pmatrix} \end{pmatrix} =\begin{pmatrix} 5 & 6 & 10 & 12 \\ 7 & 8 & 14 & 16 \\ 15 & 18 & 20 & 24 \\ 21 & 24 & 28 & 32 \end{pmatrix}$

2. Beispiel

$\begin{pmatrix} 1 & 3 & 2 \\ 1 & 0 & 0 \\ 1 & 2 & 2 \end{pmatrix} \otimes \begin{pmatrix} 0 & 5 \\ 5 & 0 \\ 1 & 1 \end{pmatrix} = \begin{pmatrix} 1 \cdot \begin{pmatrix} 0 & 5 \\ 5 & 0 \\ 1 & 1 \end{pmatrix} & 3 \cdot \begin{pmatrix} 0 & 5 \\ 5 & 0 \\ 1 & 1 \end{pmatrix} & 2 \cdot \begin{pmatrix} 0 & 5 \\ 5 & 0 \\ 1 & 1 \end{pmatrix} \\\\ 1 \cdot \begin{pmatrix} 0 & 5 \\ 5 & 0 \\ 1 & 1 \end{pmatrix} & 0 \cdot \begin{pmatrix} 0 & 5 \\ 5 & 0 \\ 1 & 1 \end{pmatrix} & 0 \cdot \begin{pmatrix} 0 & 5 \\ 5 & 0 \\ 1 & 1 \end{pmatrix} \\\\ 1 \cdot \begin{pmatrix} 0 & 5 \\ 5 & 0 \\ 1 & 1 \end{pmatrix} & 2 \cdot \begin{pmatrix} 0 & 5 \\ 5 & 0 \\ 1 & 1 \end{pmatrix} & 2 \cdot \begin{pmatrix} 0 & 5 \\ 5 & 0 \\ 1 & 1 \end{pmatrix} \end{pmatrix} =\begin{pmatrix} 0 & 5 & 0 & 15 & 0 & 10 \\ 5 & 0 & 15 & 0 & 10 & 0 \\ 1 & 1 & 3 & 3 & 2 & 2 \\ 0 & 5 & 0 & 0 & 0 & 0 \\ 5 & 0 & 0 & 0 & 0 & 0 \\ 1 & 1 & 0 & 0 & 0 & 0 \\ 0 & 5 & 0 & 10 & 0 & 10 \\ 5 & 0 & 10 & 0 & 10 & 0 \\ 1 & 1 & 2 & 2 & 2 & 2 \end{pmatrix}$

Eigenschaften

Das Kronecker-Produkt ist nicht kommutativ. Das heißt im Allgemeinen gilt

$A\otimes B\neq B\otimes A$

Es gibt jedoch Permutationsmatrizen P,Q so dass gilt

$A\otimes B=P(B\otimes A)Q$

Sind dabei A und B quadratisch so kann P = Q^T gewählt werden.

Das Kronecker-Produkt ist bilinear. Das heißt

$A\otimes (B+C)=A\otimes B+A\otimes C$

$(B+C)\otimes A=B\otimes A+C \otimes A$

$\lambda (A\otimes B)=(\lambda A)\otimes B=A\otimes (\lambda B)$

Das Kronecker-Produkt ist assoziativ. Das heißt

$A\otimes(B\otimes C)=(A\otimes B)\otimes C$

Für die Transposition gilt

$(A \otimes B)^T = A^T \otimes B^T$.

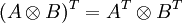Sind A und B quadratische Matrizen so gilt für die Spur

$\mathrm{Spur}(A \otimes B) = \mathrm{Spur}(A) \cdot \mathrm{Spur}(B)$.

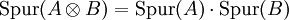Für den Rang gilt

$\mathrm{Rang}(A \otimes B) = \mathrm{Rang}(A) \cdot \mathrm{Rang}(B)$.

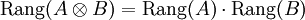Ist A eine 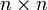$n\times n$ und B eine 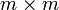$m\times m$ Matrix so gilt für die Determinante

$\det(A\otimes B)={\det}^m (A) \, {\det}^n(B)$.

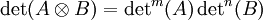Sind 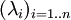$(\lambda_i)_{i=1..n}\,$ die Eigenwerte von A und 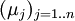$(\mu_j)_{j=1..n}\,$ die Eigenwerte von B dann gilt

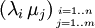$(\lambda_i \, \mu_j)_{i=1..n \atop j=1..m}$ sind die Eigenwerte von $A\otimes B$.

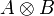Sind A,B invertierbar so ist

$(A\otimes B)^{-1}=A^{-1} \otimes B^{-1}$.

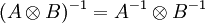Sind die (komplexen) Matrizen A,B,C und D mit den Dimensionen

• $A : m \times n$
• $B : p \times q$
• $C : n \times r$
• $D : q \times s$

gegeben, so gilt ^[1]

$AC\otimes BD=(A\otimes B)(C\otimes D)$.

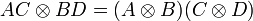Matrixgleichung

Es seien die Matrizen 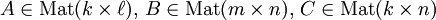$A\in\mathrm{Mat}(k\times\ell),\, B\in\mathrm{Mat}(m\times n),\, C\in\mathrm{Mat}(k\times n)$ gegeben

und eine Matrix 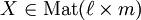$X\in\mathrm{Mat}(\ell\times m)$ gesucht so dass 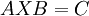$AXB=C\,$ gilt. Nun gilt folgende Äquivalenz:

$AXB=C \iff (B^T\otimes A)\, \operatorname{vec}(X)=\operatorname{vec}(C)$

Hierbei steht 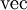$\operatorname{vec}$ für die spaltenweise Vektorisierung einer Matrix zu einem Spaltenvektor.

Sind 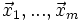$\vec{x}_1,...,\vec{x}_m$ die Spalten der Matrix 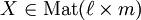$X\in\mathrm{Mat}(\ell\times m)$ so ist 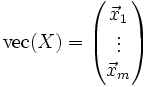$\operatorname{vec}(X)=\begin{pmatrix} \vec{x}_1 \\ \vdots \\ \vec{x}_m \end{pmatrix}$ ein Spaltenvektor der Länge $\ell\cdot m$.

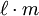Analog ist 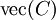$\operatorname{vec}(C)$ ein Spaltenvektor der Länge $m\cdot n$.

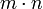Hat man den Vektor 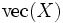$\operatorname{vec}(X)$ ermittelt so ergibt sich daraus unmittelbar die zugehörige, isomorphe Matrix $X\in\mathrm{Mat}(\ell\times m)$.

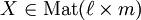Beweis der Äquivalenz

Es ist $AXB=C \iff AX\left(\vec{b}_1,...,\vec{b}_n\right)=\left(\vec{c}_1,...,\vec{c}_n\right) \iff AX \vec{b_i}=\vec{c_i} \iff \begin{pmatrix} AX \vec{b}_1 \\ \vdots \\ AX \vec{b}_n \end{pmatrix}=\operatorname{vec}(C)$

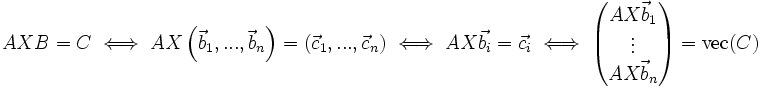
Dabei ist $\begin{pmatrix} A(\vec{x}_1,...,\vec{x}_m) \vec{b}_1 \\ \vdots \\ A(\vec{x}_1,...,\vec{x}_m) \vec{b}_n \end{pmatrix} =\begin{pmatrix} A(b_{11}\vec{x}_1+...+b_{m1}\vec{x}_m) \\ \vdots \\ A(b_{1n}\vec{x}_1+...+b_{mn}\vec{x}_m) \end{pmatrix} =\begin{pmatrix} A\, b_{11} & \cdots & A\, b_{m1} \\ \vdots & \ddots & \vdots \\ A\, b_{1n} & \cdots & A\, b_{mn}\end{pmatrix} \begin{pmatrix} \vec{x}_1 \\ \vdots \\ \vec{x}_m \end{pmatrix}=(B^T\otimes A)\, \operatorname{vec}(X)$

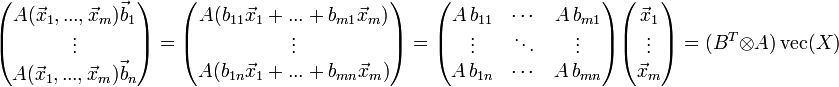
Gleichungssystem mit Matrixkoeffizienten

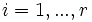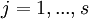Für $i=1,...,r\,$ und $j=1,...,s\,$ seien die Matrizen 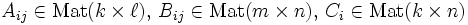$A_{ij}\in\mathrm{Mat}(k\times\ell),\, B_{ij}\in\mathrm{Mat}(m\times n),\, C_i\in\mathrm{Mat}(k\times n)$ gegeben.

Gesucht sind die Matrizen 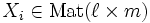$X_i\in\mathrm{Mat}(\ell\times m)$, welche das Gleichungssystem

$\begin{bmatrix} A_{11} X_1 B_{11}+...+A_{1s} X_s B_{1s} & = & C_1 \\ & \vdots & \\ A_{r1} X_1 B_{r1}+...+A_{rs} X_s B_{rs} & = & C_r \\ \end{bmatrix}$

lösen. Diese Aufgabenstellung ist äquivalent zum Lösen des Gleichungssystems

$\begin{pmatrix} B_{11}^T \otimes A_{11} & \cdots & B_{1s}^T \otimes A_{1s} \\ \vdots & \ddots & \vdots \\ B_{r1}^T \otimes A_{r1} & \cdots & B_{rs}^T \otimes A_{rs} \\ \end{pmatrix} \begin{pmatrix} \operatorname{vec} \, X_1 \\ \vdots \\ \operatorname{vec} \, X_s \end{pmatrix} =\begin{pmatrix} \operatorname{vec} \, C_1 \\ \vdots \\ \operatorname{vec} \, C_r \end{pmatrix}$

Weitere Anwendungen

Das Kronecker-Produkt wird beispielsweise in der verallgemeinerten linearen Regressionsanalyse verwendet, um eine Kovarianzmatrix von korrelierten Störgrößen zu konstruieren. Man erhält hier etwa eine blockdiagonale Zellnermatrix.

Zudem braucht man das Kronecker-Produkt in der Quantenmechanik um Systeme mit mehreren Teilchen, die ein beidseitig beschränktes Spektrum besitzen, zu beschreiben. Zustände mehrerer Teilchen sind dann Kroneckerprodukte der Einteilchenzustände. Im Falle eines unbeschränkten Spektrums bleibt nur die algebraische Struktur eines Kronecker-Produktes erhalten, da dann keine Darstellung durch Matrizen existiert.

Zusammenhang mit Tensorprodukten

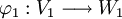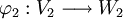Gegeben seien zwei lineare Abbildungen $\varphi_1:V_1\longrightarrow W_1$ und $\varphi_2:V_2\longrightarrow W_2$ zwischen endlich dimensionalen Vektorräumen. Dann gibt es immer genau eine lineare Abbildung

$\varphi_1\otimes\varphi_2:V_1\otimes V_2\longrightarrow W_1\otimes W_2$

zwischen den Tensorprodukten mit $\varphi_1\otimes \varphi_2(v_1\otimes v_2)=\varphi_1(v_1)\otimes \varphi_2(v_2)$.

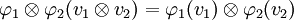Wenn wir auf den Vektorräumen V₁,W₁,V₂ und W₂ je eine Basis auswählen, so können wir der Abbildung 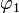$\varphi_1$ ihre Darstellungsmatrix A zuordnen, B sei die Darstellungsmatrix von 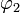$\varphi_2$. Das Kronecker-Produkt 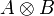$A\otimes B$ der Darstellungsmatrizen ist nun genau die Darstellungsmatrix der tensorierten Abbildung $\varphi_1\otimes\varphi_2$.

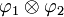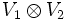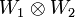Damit dies funktioniert, müssen aber die Basen auf $V_1\otimes V_2$ und $W_1\otimes W_2$ richtig gewählt werden: Wenn 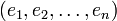$(e_1,e_2,\ldots, e_n)$ die ausgewählte Basis von V₁ und 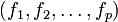$(f_1,f_2,\ldots, f_p)$ die Basis von V₂ gegeben ist, so nehmen wir 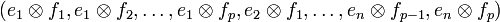$(e_1\otimes f_1, e_1\otimes f_2, \ldots, e_1\otimes f_p, e_2\otimes f_1, \ldots, e_n\otimes f_{p-1}, e_n\otimes f_p)$ als Basis für das Tensorprodukt 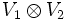$V_1\otimes V_2$. Analog für $W_1\otimes W_2$.

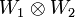Historisches

Das Kronecker-Produkt ist nach Leopold Kronecker benannt, weil er es anscheinend als erster definierte und verwendete. Früher wurde das Kronecker-Produkt manchmal Zehfuss-Matrix genannt, nach Johann Georg Zehfuss.

Weblinks

• PlanetMath Artikel: Kronecker Product
• MathWorld: Matrix Direct Product

Quellen

1. ↑ Steeb, Willi Hans: Kronecker Product of Matrices and Applications. BI-Wiss.Verlag, 1991, ISBN 3-411-14811-X, S.16