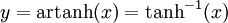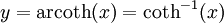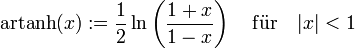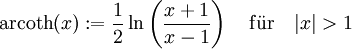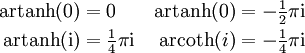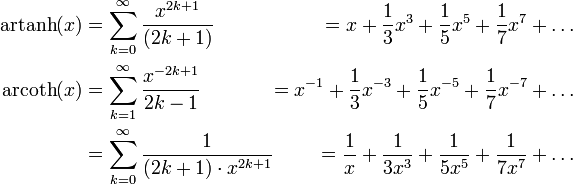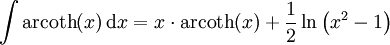- Areacotangens Hyperbolicus
-
Areatangens Hyperbolicus und Areakotangens Hyperbolicus sind die Umkehrfunktionen von Tangens Hyperbolicus und Kotangens Hyperbolicus und damit Area-Funktionen.
Schreibweisen:
Letztere wird seltener benutzt, um die Verwechselung mit dem Kehrwert des hyperbolischen (Ko)Tangens zu vermeiden. Es ist
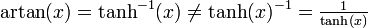 .
.Inhaltsverzeichnis
Definitionen
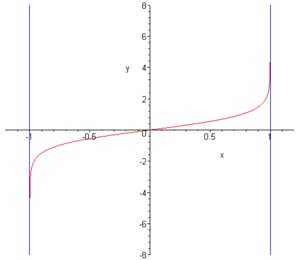
Areatangens Hyperbolicus:
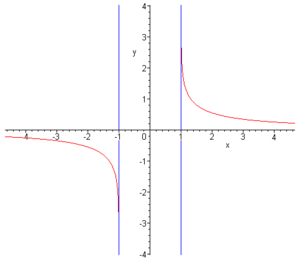
Areakotangens Hyperbolicus:
Geometrische Definitionen
Geometrisch lässt sich der Areatangens Hyperbolicus durch die Fläche in der Ebene darstellen, welche die Verbindungsstrecke zwischen dem Koordinatenursprung (x,y) = (0,0) und der Hyperbel x2 − y2 = 1 überstreicht: Es seien
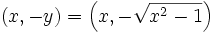 und
und 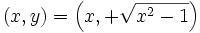 Start- und Endpunkt auf der Hyperbel, dann wird von der Verbindungsstrecke die Fläche
Start- und Endpunkt auf der Hyperbel, dann wird von der Verbindungsstrecke die Fläche  überstrichen.
überstrichen.Spezielle Werte
Eigenschaften
Areatangens Hyperbolicus Areakotangens Hyperbolicus Definitionsbereich − 1 < x < 1 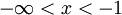
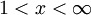
Wertebereich 
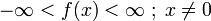
Periodizität keine keine Monotonie streng monoton steigend streng monoton fallend Symmetrien ungerade Funktion:
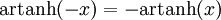
ungerade Funktion:
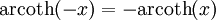
Asymptote 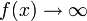 für
für 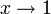
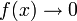 für
für 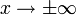
Nullstellen x = 0 keine Sprungstellen keine keine Polstellen 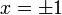
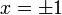
Extrema keine keine Wendepunkte x = 0 keine Reihenentwicklungen
Taylor- und Laurent-Reihen der beiden Funktionen sind
Ableitungen
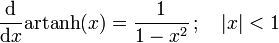 .
.
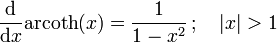 .
.
Integrale
Die Stammfunktionen lauten:
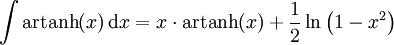 .
.
Siehe auch
Weblinks
- Eric W. Weisstein: Inverse Hyperbolic Tangent und Inverse Hyperbolic Cotengent auf MathWorld
Primäre trigonometrische Funktionen
Sinus und Kosinus | Tangens und Kotangens | Sekans und KosekansUmkehrfunktionen (Arkusfunktionen)
Arkussinus und Arkuskosinus | Arkustangens und Arkuskotangens | Arkussekans und ArkuskosekansHyperbelfunktionen
Sinus Hyperbolicus und Kosinus Hyperbolicus | Tangens Hyperbolicus und Kotangens Hyperbolicus | Sekans Hyperbolicus und Kosekans HyperbolicusAreafunktionen
Areasinus Hyperbolicus und Areakosinus Hyperbolicus | Areatangens Hyperbolicus und Areakotangens Hyperbolicus | Areasekans Hyperbolicus und Areakosekans Hyperbolicus
Wikimedia Foundation.